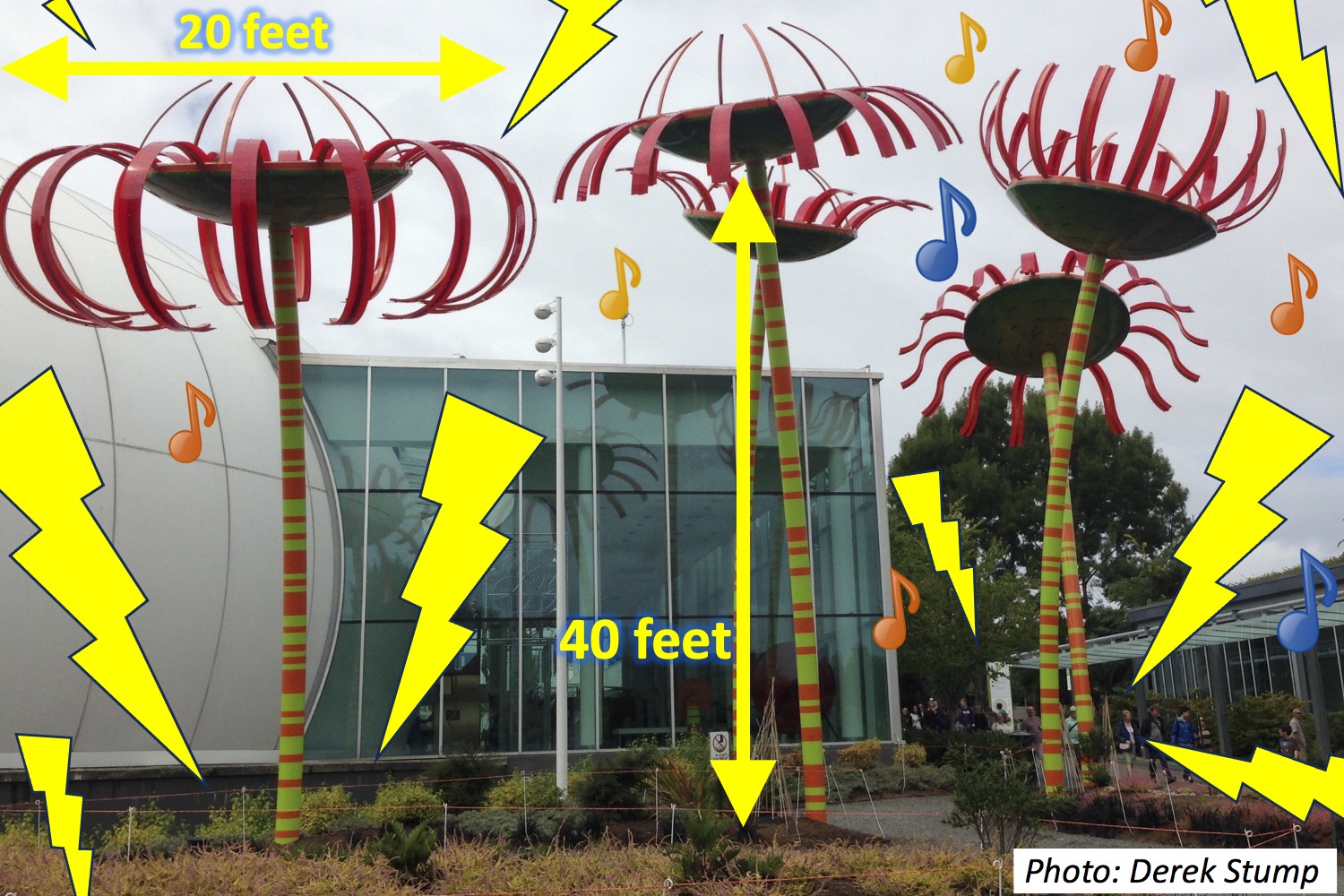
These wacky flower sculptures look like a picture from a Dr. Seuss book. Built by artist Dan Corson, they stand 40 feet tall, each flower center has little solar panels on it. Best of all, the flowers sing: they have sensors that tell them when people walk near them, causing the flowers to play musical notes. So even though you can’t smell these flowers, they’re making up for it by doing a lot of other things!
Wee ones: If you make all 5 flowers sing, what numbers would you say to count them?
Little kids: If you swing from the petals and grab the 2nd one, then the 4th one, then the 6th…which petal do you grab next? Bonus: Each flower is 20 feet across! If you lay across the top of it, how many feet wider than you would it be? (Find your height to the nearest foot.)
Big kids: If the sun is out from 6:00 in the morning until 8:00 at night, how many hours do the flowers collect sunlight that day? Bonus: Each flower has 3 circles of solar panels: 10 in the innermost ring, 16 in the next ring, and 23 in the outside ring around them. How many panels is that on each flower?
The sky’s the limit: If there are 5 flowers and by waving your arms you make 3 of them sing, how many different possible trios of flowers could you get to sing? (Don’t worry about the order, just which ones are in the set.)
Answers:
Wee ones: 1, 2, 3, 4, 5.
Little kids: The 8th petal. Bonus: Different for everyone…subtract your height in feet from 20.
Big kids: 14 hours. Bonus: 49 panels.
The sky’s the limit: There are 10 trios. Choosing 3 of them gives the same number of sets as leaving out 2, so you can just count up the possible pairs to leave out. If they’re lettered A, B, C, D and E, you have 4 pairs with A (AB, AC, AD, AE), then 3 new pairs using B (BC, BD, BE), then 2 pairs with C (CD, CE), and finally DE. That gives us 4+3+2+1=10.